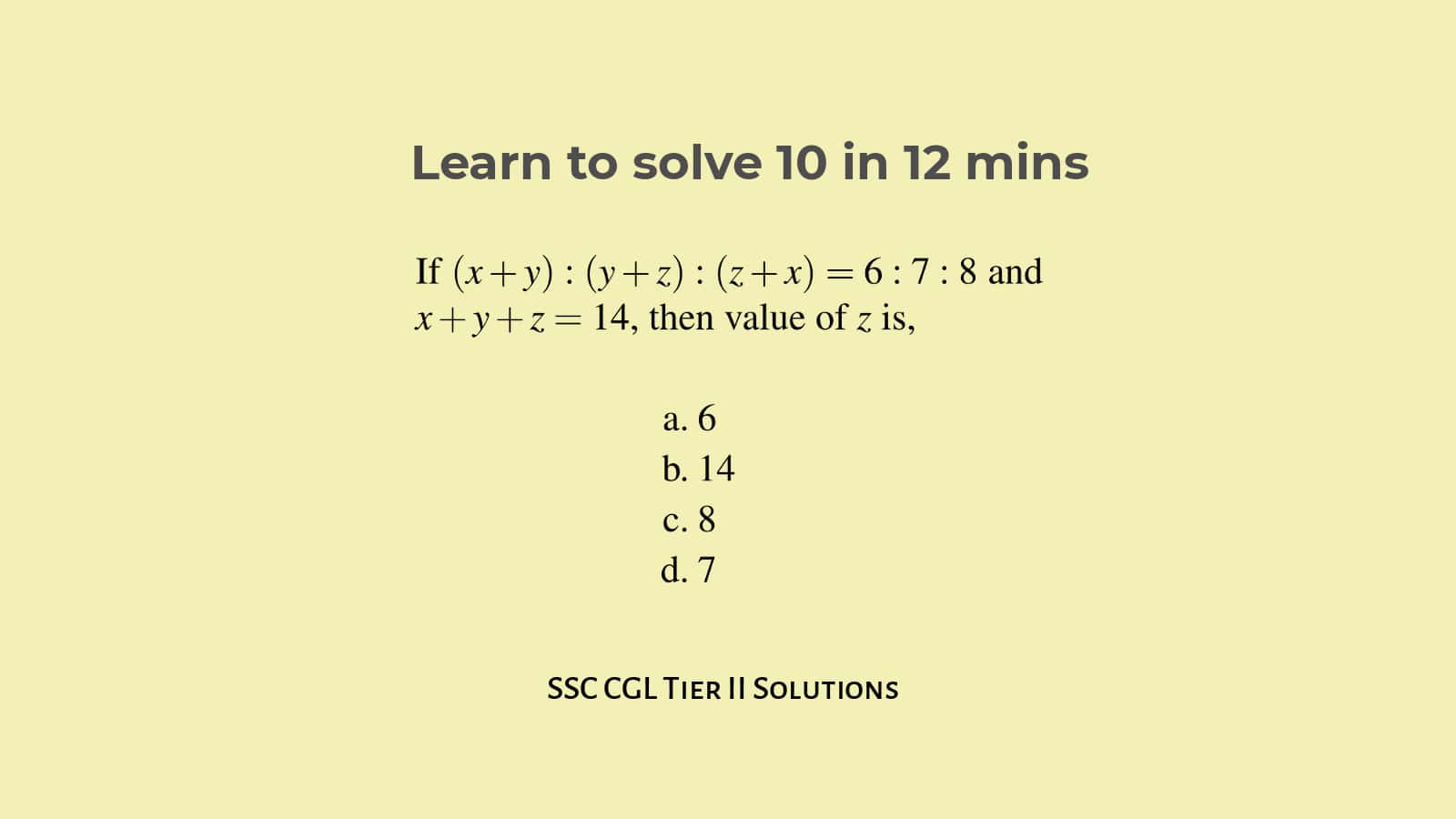
Algebra questions for SSC CGL Tier 2 Set 1 Solved Quickly

Submitted by Atanu Chaudhuri on Mon, 17/10/2016 - 14:45

Algebra Questions for SSC CGL Quick Solutions Set 1

Learn to solve 10 algebra questions for SSC CGL Tier 2 in 12 minutes. Each question is solved taking as few steps as possible using advanced techniques.

If you have not yet taken this test take it first at,

SSC CGL Tier II level question set 1 on Algebra 1.

Quick solutions to 10 Algebra questions for SSC CGL Set 1 - answering time was 12 mins

Q1. Find the maximum value of the expression $(p^2 +7p+13)^{-1}$.

$\displaystyle\frac{5}{7}$
$\displaystyle\frac{4}{3}$
$20$
$\displaystyle\frac{3}{7}$

Solution 1 - Problem analysis

We are required to find the maximum value of the given expression,

$E=(p^2 +7p+13)^{-1}$

$\hspace{5mm}=\displaystyle\frac{1}{p^2 +7p+13}$

$\hspace{5mm}=\displaystyle\frac{1}{E_1}$

At the minimum value of the expression $E_1$ in the denominator, the given expression $E$ reaches its maximum value.

So we can rephrase the problem as,

Find the minimum value of the expression $(p^2 +7p+13)$.

Solution 1 - Problem solving execution using Maxima minima technique

The problem now has been transformed to a straightforward application of maxima minima technique where we express the target quadratic equation in two parts, the main part being a square of a sum. Let us see how.

$E_1=p^2 +7p+13$

$\hspace{7mm}=\left(p+\displaystyle\frac{7}{2}\right)^2 - \displaystyle\frac{49}{4} + 13$

$\hspace{7mm}=\left(p+\displaystyle\frac{7}{2}\right)^2 + \displaystyle\frac{3}{4}$.

The expression $E_1$ will reach its minimum value only when the square of sum term is zero. Thus the minimum value of $E_1$ is, $\displaystyle\frac{3}{4}$.

Finally then the desired maximum value of,

$E=\displaystyle\frac{1}{E_1}=\frac{4}{3}$.

Answer: Option b: $\displaystyle\frac{4}{3}$.

Key concepts used: Realizing that maximum of the inverse expression is actually the minimum of the expression in the denominator, maxima minima technique is used directly on the denominator expression -- key information discovery.

Q2. If $n=7+3\sqrt{5}$, then the value of $\left(\sqrt{n} + \displaystyle\frac{1}{\sqrt{n}}\right)$ is,

$\displaystyle\frac{5}{7}$
$\displaystyle\frac{7}{12}$
$\displaystyle\frac{9+\sqrt{5}}{2\sqrt{2}}$
$\displaystyle\frac{\sqrt{5}}{2\sqrt{2}}$

Solution 2 - Problem analysis

This is a standard surd problem with target expression in the form of sum of inverses where we will need to rationalize the inverse term to find the desired value of the target expression. But the main hurdle we face first is the presence of $\sqrt{n}$ in the target expression, where the given expression is in terms of $n$.

This necessitates transformation of the surd expression on the RHS of the given equation as a square of sum so that we can get the value of $\sqrt{n}$ as a surd expression that we can deal with. This is direct application of deductive reasoning.

The problem now is transformed to,

Transform the surd expression $7+3\sqrt{5}$ as a square of sum surd expression.

According to the Coefficient 2 of a surd term principle, to transform a surd expression to a square of sum surd expression, the first thing we look for is a $2$ as a factor of the coefficient of the surd term which is not there in our surd expression. So as a part of Conversion to a square of sum surd expression technique, we multiply the surd expression by $2$ and also divide it by $2$,

$n=7+3\sqrt{5}$

$\hspace{5mm}=\displaystyle\frac{1}{2}(14+6\sqrt{5})$

$\hspace{5mm}=\displaystyle\frac{1}{2}(3 +\sqrt{5})^2$,

Or, $\sqrt{n}=\displaystyle\frac{1}{\sqrt{2}}(3 + \sqrt{5})$.

Conversion to square of sum surd expression technique

Acknowldging the fact that not all two term surd expressions can be converted to a square of another two term surd expression, oftentimes ability to convert a two term surd expression to a square of a second two term surd expression turns out to be the key to solving an especially knotty problem.

Thus, apart from Surd rationalization technique, we consider this conversion to square of sum surd expression technique as the second most important concept in the area of Surd Simplification.

The following are the steps,

Step 1: In the first step of this technique we look for $2$ in the coefficient of the surd term. This action follows the principle of coefficient $2$ of surd middle term.

Step 2: If we find $2$ as a factor of the coefficient of the surd term, we assume the surd term to be equivalent to $2ab$ and integer term equivalent to $a^2+b^2$. In case the second equivalence is satisfied, the given surd expression can be transformed to $(a+b)^2$, where one of $a$ or $b$ is a surd. Otherwise the given surd expression can't be transformed as a square of a second two term surd expression.

Step 3: If we do not find a $2$ as a factor of the coefficient of the surd term we introduce it by multiplying and dividing the surd expression by $2$ and then follow Step 2.

Let us show how the two variations of the technique works by an example for each case.

Problem Example 1

Transform $11+6\sqrt{2}$ as a square of a second two term surd expression.

Solution example 1

$11+6\sqrt{2}=(3)^2+2\times{3}\times{\sqrt{2}}+ (\sqrt{2})^2$

$=(3+\sqrt{2})^2$.

Problem example 2

Transform $2+\sqrt{3}$ as a square of two term surd expression.

Solution example 2

As the given surd expression does not have a $2$ as a factor of coefficient of the surd term, we introduce it by multiplying and dividing the expression by $2$,

$2+\sqrt{3}=\displaystyle\frac{1}{2}\left(4+2\sqrt{3}\right)$

$=\displaystyle\frac{1}{2}\left(1+2.1.\sqrt{3}+(\sqrt{3})^2\right)$

$=\displaystyle\frac{1}{2}\left(1+\sqrt{3}\right)^2$

Let us see why this technique works.

Mechanism of conversion to square of sum surd expression technique

Let us assume the original two term surd expression to be converted as,

$(a^2+b^2) + 2ab=(a+b)^2$, where $(a^2+b^2)$ is the integer term and $2ab$ is the surd term. This assumption is valid as, each of the two $a$ and $b$ being squared in $a^2+b^2$, the result is always an integer. And the surd among $a$ and $b$ remains unchanged as a surd in the second term $2ab$ which then is always the surd term. Additionally this surd term plays its important role as the middle term in the expansion of $(a+b)^2$.

This is why we look for $2$ in the surd term and try to equate $a^2+b^2$ with the integer term.

Solution 2 - Problem solving execution second stage

So,

$\displaystyle\frac{1}{\sqrt{n}}=\sqrt{2}\displaystyle\frac{1}{3+\sqrt{5}}$

$=\sqrt{2}\left(\displaystyle\frac{3-\sqrt{5}}{4}\right)$, rationalization multiplying numerator and denominator by $3 - \sqrt{5}$

$=\displaystyle\frac{3-\sqrt{5}}{2\sqrt{2}}$.

Finally,

$\left(\sqrt{n} + \displaystyle\frac{1}{\sqrt{n}}\right) = \displaystyle\frac{9+\sqrt{5}}{2\sqrt{2}}$.

Answer: Option c : $\displaystyle\frac{9+\sqrt{5}}{2\sqrt{2}}$.

Key concepts used: Key information discovery -- deductive reasoning -- input transformation to a square of sum -- conversion to square of sum surd expression technique -- surd rationalization -- Principle of coefficient 2 of surd middle term -- simplification.

Q3. If $x=20$, $y=19$, the value of $\displaystyle\frac{x^2+y^2+xy}{x^3-y^3}$ is,

$35$
$1$
$324$
$365$

Solution 3 - Problem analysis and solving

On examining the target expression we find the numerator to be a factor of the denominator,

$x^3-y^3=(x-y)(x^2+xy+y^2)$.

Thus the target expression reduces to,

$E=\displaystyle\frac{x^2+y^2+xy}{x^3-y^3}$

$\hspace{5mm}=\displaystyle\frac{1}{x-y}$

$\hspace{5mm}=\displaystyle\frac{1}{20-19}$

$\hspace{5mm}=1$.

Answer: Option b: 1.

Key concepts used: Factors of sum of cubes -- key pattern identification -- simplification.

Q4. If $p + \displaystyle\frac{2p}{3} + \displaystyle\frac{p}{2} +\displaystyle\frac{p}{7}=97$, then the value of $p$ is,

Solution 4 - Problem analysis and solving

Essentially this problem turns out to be an evaluation of sum of fractions,

$p + \displaystyle\frac{2p}{3} + \displaystyle\frac{p}{2} +\displaystyle\frac{p}{7}=97$,

Or, $p\left(1 + \displaystyle\frac{2}{3} + \displaystyle\frac{1}{2} +\displaystyle\frac{1}{7}\right)=97$,

Or, $p\left(\displaystyle\frac{42+ 28+21+6}{42}\right)=97$,

Or, $p\left(\displaystyle\frac{97}{42}\right)=97$,

Or, $p=42$.

Answer: Option d: 42.

Key concepts used: Fraction sum expression simplification.

Q5. If $p=1+\sqrt{2}+\sqrt{3}$, then $\left(p+\displaystyle\frac{1}{p-1}\right)$ is,

$1 +2\sqrt{3}$
$2\sqrt{3}-1$
$2+\sqrt{3}$
$3+\sqrt{2}$

Solution 5 - Problem analysis

Following End state analysis we compare the target expression with the given expression to find that by transforming both the target and given expression in terms of $p-1$ we will be able to apply the sum of inverses principle to the pair of expressions.

Solution 5 - Problem solving execution

Transforming the target expression first we get,

$E=\left(1+(p-1)+\displaystyle\frac{1}{(p-1)}\right)$

$\hspace{5mm}=1+\left(q+\displaystyle\frac{1}{q}\right)$, where $q=p-1$

$\hspace{5mm}=1+E_1$, where $E_1$ is a pure sum of inverses expression.

Similarly transforming the given expression we have,

$p=1+\sqrt{2}+\sqrt{3}$,

Or, $p-1=q=\sqrt{3}+\sqrt{2}$.

And $\displaystyle\frac{1}{q}=\frac{1}{\sqrt{3}+\sqrt{2}}$

Rationalizing the surd expression on the RHS we get,

$\displaystyle\frac{1}{q}=\sqrt{3}-\sqrt{2}$.

So the sum of inverses is,

$q+\displaystyle\frac{1}{q}=E_1=2\sqrt{3}$.

Finally then the target expression,

$E=1+E_1=1+2\sqrt{3}$.

Answer: Option a: $1+2\sqrt{3}$.

Key concepts used: End state analysis -- deductive reasoning -- pattern identification -- input and output expression transformation to make the two expressions in terms of same variable $p-1$ -- principle of inverses -- surd rationalization -- simplification.

Q6. If $(x+y) : (y+z) : (z+x) = 6 : 7 : 8$ and $x+y+z=14$, then value of $z$ is,

Solution 6 - Problem analysis

The given expression is equivalent to a three variable ratio of $p=(x+y)$, $q=(y+z)$ and $r=(z+x)$ so that $p:q:r=6a:7a:8a$, where the values $6a$, $7a$ and $8a$ are presumed to be the actual values of the three ratio variables obtained by re-introducing the canceled out HCF of the actual values of the three variables $p$, $q$ and $r$. This is appplication of basic ratio concepts.

Adding the values of the three variables following principle of collection of friendly terms we have now,

$(p+q+r)=a(6+7+8)=21a$,

Or, $2(x+y+z)=21a$,

Or, $28=21a$,

Or, $a=\displaystyle\frac{4}{3}$.

From the given ratio and value of the sum $x+y+z=14$ we are able to find out the value of canceled out HCF so that we will now be able to manipulate the actual values of three ratio variables suitably.

Solution 6 - Problem solving execution

As we need $z$ we will add up the two parts $q=(y+z)$ and $r=(z+x)$ that have $z$ as common. Our objective is to replace the resulting second portion $x+y$ by $z$ again from the value of $x+y+z$. Adding $q$ and $r$ we have then,

$(x+y+2z)=(7+8)a=15a$,

Or, $(14-z) +2z=20$, as $a=\displaystyle\frac{4}{3}$

Or, $z=6$.

Answer: Option a : 6.

Key concepts used: Basic ratio concepts -- re-introduction of canceled out HCF for dealing with actual values of the ratio variable parts -- deductive reasoning -- Principle of collection of friendly terms -- simplification.

Q7. If $x=\displaystyle\frac{\sqrt{2}+1}{\sqrt{2}-1}$ and $xy=1$ find the value of $\displaystyle\frac{2x^2+3xy+2y^2}{2x^2-3xy+2y^2}$.

$\displaystyle\frac{71}{65}$
$\displaystyle\frac{7}{12}$
$\displaystyle\frac{65}{71}$
$\displaystyle\frac{72}{7}$

Solution 7 - Problem analysis and strategy formulation

On examining the two expressions in the numerator and denominator of the target expression we find that both of these two can be expressed in terms of $(x+y)^2$ and $xy$. So if we find out just the value of $(x+y)^2$ from the given value of $x$ and $xy$, without much detailed calculation we should be able to reach the solution quickly.

This is transformation of target expression in terms of easy to evaluate intermediate expressions and then with the value of the intermediate expressions evaluating the target expression without using the value of the elementary variables for evaluation avoiding detailed and time consuming calculation. This is a powerful general technique employed often in simplifification. We name this technique as Intermediate expression use technique. Use of this technique depends heavily on useful pattern identification skills.

Solution 7 - Problem solving execution

From $xy=1$, we have $y=\displaystyle\frac{1}{x}=\displaystyle\frac{\sqrt{2}-1}{\sqrt{2}+1}$.

So,

$x+y=\displaystyle\frac{\sqrt{2}+1}{\sqrt{2}-1} + \displaystyle\frac{\sqrt{2}-1}{\sqrt{2}+1}$

$=(\sqrt{2}+1)^2 + (\sqrt{2}-1)^2$

$=6$, a quite simplified result.

Solution 7 - Problem solving execution second stage

The target expression can be transformed as,

$E=\displaystyle\frac{2x^2+3xy+2y^2}{2x^2-3xy+2y^2}$

$\hspace{5mm}=\left(\displaystyle\frac{2(x+y)^2-xy}{2(x+y)^2-7xy}\right)$

$\hspace{5mm}=\left(\displaystyle\frac{72-1}{72-7}\right)$

$\hspace{5mm}=\displaystyle\frac{71}{65}$.

Answer: Option a: $\displaystyle\frac{71}{65}$.

Key concepts used: Pattern identification -- identifying intermediate expressions to be used in evaluating target expression -- surd simplification in evaluation of intermediate expression -- target expression transformation in terms of intermediate expressions -- substitution and simplification -- intermediate expression use technique.

Q8. If $x=\displaystyle\frac{a+b}{1-ab}$ and $y=\displaystyle\frac{a-b}{1+ab}$ then the value of $\displaystyle\frac{x+y}{1-xy}$ is,

$\displaystyle\frac{a}{1+b^2}$
$\displaystyle\frac{2a}{1+b}$
$\displaystyle\frac{2a}{1-a^2}$
$\displaystyle\frac{2a}{1+a^2}$

Solution 8 - Problem analysis:

On a quick analysis we have found no special pattern to cut short the problem except the important fact that, both the denominator and numerator of the target expression will have fraction expressions, denominators of each of which will have the common expression,

$(1-a^2b^2)$.

This will make the denominators of the two fractions in the numerator and denominator to cancel out easing the situation significantly.

Solution 8 - Problem solving execution

From the given values of $x$ and $y$ the sum of these two is,

$(x+y)=\displaystyle\frac{(a+b)(1+ab)+(a-b)(1-ab)}{1-a^2b^2}$

$=\displaystyle\frac{2a+2ab^2}{1-a^2b^2}$

$=\displaystyle\frac{2a(1+b^2)}{1-a^2b^2}$

Similarly multiplying $x$ and $y$ we have,

$xy=\displaystyle\frac{a^2-b^2}{1-a^2b^2}$,

Or, $1-xy=\displaystyle\frac{1-a^2b^2 -a^2 +b^2}{1-a^2b^2}$,

$=\displaystyle\frac{(1-a^2)(1+b^2)}{1-a^2b^2}$,

Thus the target expression evaluates to simply,

$E=\displaystyle\frac{x+y}{1-xy}$.

$=\displaystyle\frac{2a}{1-a^2}$, where $\displaystyle\frac{1+b^2}{1-a^2b^2}$ cancels out.

Answer: Option c: $\displaystyle\frac{2a}{1-a^2}$.

Key concepts used: Pattern identification -- efficient simplification.

Q9. If $x+\displaystyle\frac{1}{x}=-2$, then the value of $x^{2n+1}+\displaystyle\frac{1}{x^{2n+1}}$ where $n$ is a positive integer is,

$0$
$2$
$-5$
$-2$

Solution 9 - Problem analysis

Usually when given equation is in terms of unit power of $x$ and target expression is in terms of power $n$ of variable $x$ we should be able to evaluate the actual value of $x$ from the given equation.

Solution 9 - Problem solving execution

We have the given expression,

$x+\displaystyle\frac{1}{x}=-2$

Squaring both sides and rearranging,

$x^2- 2+\displaystyle\frac{1}{x^2}=0$,

Or, $\left(x-\displaystyle\frac{1}{x}\right)^2=0$,

Or, $\left(x-\displaystyle\frac{1}{x}\right)=0$.

So adding this result with $x+\displaystyle\frac{1}{x}=-2$ we get,

$2x=-2$,

Or, $x=-1$.

Thus target expression,

$E=x^{2n+1}+\displaystyle\frac{1}{x^{2n+1}}$

$=(-1)^{2n+1} + \displaystyle\frac{1}{(-1)^{2n+1}}$

$=-2$

Answer: Option d: -2.

Key concepts used: Deductive reasoning -- principle of inverses -- evaluating actual value of $x$ to get the value of sum of inverses in unknown odd power of $x$.

Q10. If $x=5^{n-1} +5^{-n-1}$ where $n$ is real, the minimum value of $x$ is,

$10$
$\displaystyle\frac{5}{2}$
$2$
$\displaystyle\frac{2}{5}$

Solution 10 - Problem analysis and first stage solving

Identifying that the RHS of the given expression conforms to a sum of inverses expression, we take the first step to convert it to a pure sum of inverses expression,

$x=5^{n-1} +5^{-n-1}$

$\hspace{5mm}=\displaystyle\frac{1}{5}\left(5^n+\displaystyle\frac{1}{5^n}\right)$

$\hspace{5mm}=\displaystyle\frac{1}{5}\left(E\right)$, where $x$ will be minimum when expression $E=5^n+\displaystyle\frac{1}{5^n}$ is minimum.

Solution 10 - Finding the minimum of a sum of inverses expression

Let us assume $5^n=p$ to reduce one stage of apparent complexity. As $n$ has real values, $p$ will also have real values. We now have to find the minimum of,

$p+\displaystyle\frac{1}{p}$ where $p$ is real.

This is an important concept of minimum of sum of inverses.

We have,

$E=p+\displaystyle\frac{1}{p}=\displaystyle\frac{p^2+1}{p}$.

When $p \geq 1$, $p^2$ in the numerator will increase much faster than $p$ in the denominator starting from the value of $p=1$ when $E=2$. This will happen as square of any real number greater than 1 is larger than the number itself.

Thus in this range of values of $p$, minimum value of $E$ is 2.

Let us now assume again, $p=\displaystyle\frac{1}{q}$.

This transforms the expression $E$ to,

$E=p+\displaystyle\frac{1}{p}=\displaystyle\frac{1}{q} + q=q+\displaystyle\frac{1}{q}$.

Essentially the form of the sum of inverses expression doesn't change.

In this second case we consider all values of $p$ where $p \leq 1$ and $q \geq 1$.

By the same reasoning as before, the expression E will increase indefinitely from initial value 2 when $p$ goes on decreasing starting from 1. In this case also the minimum value of $E$ is then 2.

Finally then the minimum value of $E$ for all possible values of $p$ is $2$ and the desired minimum value of the given expression is, $\displaystyle\frac{2}{5}$.

Answer: Option d: $\displaystyle\frac{2}{5}$.

Key concepts used: Transformation of input expression to a pure sum of inverses expression -- Abstraction by substitution technique reducing unnecessary complexity -- variable value trend analysis to reach the minima.

Guided help on Algebra in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Algebra in Suresolv, follow the guide,

Suresolv Algebra Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Algebra in Suresolv and is up-to-date.

SSC CGL Algebra questions with solutions

You are here

SSC CGL Tier II level Solution Set 1, Algebra 1