Clock time regulates our day

Basic concepts on Clock problems
For an analog clock,
- An analog clock face is circular and the boundary line is divided into 60 small minute sections, as 60 minutes make an hour. Each fifth such small dividing mark is slightly larger indicating a 5 minute interval. Consecutive such 5 minute intervals are marked and numbered from the top right towards the bottom and then on towards top left as 1, 2, 3,...,12. These are used as hour marks. All the the three hands move along this direction of top right to bottom and then on to top left completing the circle. This direction of movement is called clockwise movement.
- The second hand moves over the 60 marks on the face, covering each small section in 1 second. In 60 seconds it makes a complete round. The movement of this second hand produces the ticking sound. As the second hand moves round the clock face making one round per minute, the minute hand moves slower and makes one round in 60 minutes or 1 hour. The slowest and smallest hand is the hour hand. It makes one round in 12 hours. In a day it makes two complete rounds.
- The hour hand moves from one number to the next (one hour mark to the next) as the minute hand makes a complete round. As one complete round represents $360^0$, each number section divides this $360^0$ into twelve equal parts of $30^0$ each. In other words, angle held by two consecutive numbers at the centre is $30^0$. Similarly each of the 60 minute sections holds an angle of one-fifth of $30^0$, that is, $6^0$ .
- Relative speed of minute and hour hand: Minute hand moves $6^0$ every minute while the hour hand moves from one hour digit to the next, that is, $30^0$ in one hour or 60 mins. So hour hand moves $\displaystyle\frac{1}{2}^0$ every minute. Thus the relative speed of minute and hour hands is, $6^0-\displaystyle\frac{1}{2}^0 = \displaystyle\frac{11}{2}^0$ every minute. With this relative speed the minute hand approaches the effectively stationary hour hand.
- Crossing of hour and minute hands: Between each two hours, the minute and hour hands coincide once, except that this happens only once between 11 and 1 at 12 am or pm, not twice. Thus, minute and hour hands coincide 11 times every 12 hours and 22 times a day.
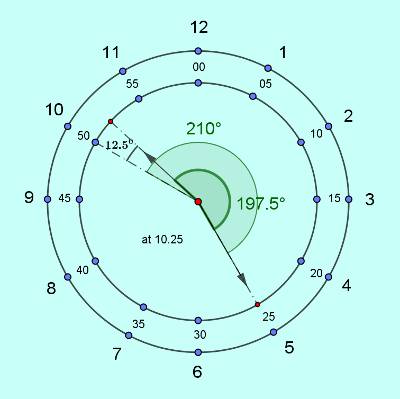
A schematic of an analog clock face at 10.25 should help to clarify the concepts,
Problem examples on Clocks
Clock Problem example 1:
At what time between 2 O’clock and 3 O’clock, will the minute and hour hands be together?
Clock Problem Solution 1:
At 2 pm or am, the hour hand is aligned exactly with 2 and the minute hand at 12. Let us assume each minute section of the clock face to be a unit of speed of the minute and hour hands.
The minute hand moves 1 unit per minute whereas the hour hand moves 5 units in 60 minutes, that is,
$\displaystyle\frac{1}{12}$ units per minute.
These are the speed of movements of the two hands. Thus the relative speed of the two hands is,
$1 - \displaystyle\frac{1}{12} = \displaystyle\frac{11}{12}$ units per minute.
This is the speed at which the minute hand approaches the effectively stationary hour hand.
At 2 pm or am, the hour hand is ahead of the minute hand by 10 units. To cover this 10 units at the relative speed, the minute hand will take a time of,
$T = 10\times{\displaystyle\frac{11}{12}} = \displaystyle\frac{120}{11} = 10\displaystyle\frac{10}{11}\text{ min }$.
So,
$\displaystyle\frac{10}{11}$ mins past 10 past 2, the two hands will coincide.
This is a problem of catching up or race.
Alternate clock problem solution 1:
At 2 pm or am, the hour hand is aligned exactly with 2 and the minute hand at 12, that is, hour hand is ahead of minute hand by $2 \times{30^0} = 60^0$.
We know, the minute hand approaches effectively stationary hour hand at a relative speed of $\displaystyle\frac{11}{2}^0$ every minute. Thus to make up or cover the lag of $60^0$, the minute hand will take,
$T = \displaystyle\frac{60^0}{\displaystyle\frac{11^0}{2}}$
$=\displaystyle\frac{120}{11}$
$= 10\displaystyle\frac{10}{11}$ mins.
This is the time when the two hands will coincide between 2 O’clock and 3 O’clock.
Clock Problem example 2:
What is the angle between the hour and minute hands at 20 minutes past 2?
Clock Problem Solution 2:
At 20 minutes past 2 the minute hand points at 4 and has moved from zero position through an angle of $6^0\times{20} = 120^0$. We know, minute hand moves $360^0$ in 60 mins and so moves $6^0$ in 1 min.
At 2, the hour hand pointed to 2 and had already moved by $60^0$, as each marked number 1 to 12 represents an angle of $360^0 \div{12} = 30^0$.
Furthermore, in 60 mins, the hour hand covers the space between two marked digits, that is, covers $30^0$. So the hour hand moves $\displaystyle\frac{1}{2}^0$ in each minute. Thus, at 2 past 20 mins it will cover in total,
$60^0 + 20\times{\displaystyle\frac{1}{2}}^0 = 70^0$.
So the required angle between the hour and the minute hands will be,
$120^0 - 70^0 = 50^0$.
The corresponding clock figure is shown below,

In this way, most of the clock problems are based on angular speeds of the minute and hour hands, or their relative speed.
